Topology is a wonderful visual source for artists. Knotted spheres, surfaces that use 4-space to avoid intersecting, planes that have no edges, a bottle that turns inside out. There is even the humble moebius strip that disguises itself as a 3-dimensional object even though it has only one edge and one side. I promise you won’t find any mathematics here. I like to say that I gather beautiful flowers from the field of Topology. Below are some notations and sketches and other ‘stuff’ lying around my studio.
In 1985 my husband and I determined to make copies of the wonderful ceramics of ancient Cyprus. We were very fortunate to be allowed to study the Cypriot collection at the British Museum. For over two years we got up close and personal with vessels over four thousand years old! 1988 we moved to a small village in the north west tip of Cyprus, where we started Triskelion Pottery. I think we did a pretty darn good job of it. I hope you enjoy looking at the photos of our work.
A third string to my bow over the years has been portrait sculpture and drawing portraits. I went to the Central School of Art in the 1970s where such conventional endeavours were seriously frowned upon in the sculpture department. But despite the heavy disapproval I have returned to portraiture again and again and remain completely intrigued by every face I see.
My husband, Ara Nigogossian, now deceased, experimented with Early Bronze Age firing methods and from this emerged a unique technique that we dubbed his Smoke Pots. Apart from saying that i find them exquisit I will let them speak for themselves.
Notebook ; from braid to knot
I was trying to figure out how to turn the braid diagram of knot 10_32 in to its knot. I believe topologists do this the other way around but I was really proud of myself for succeeding.
Braid diagrams and their knots
Blue mesh knots waiting for their time in the limelight.
Each knot has its identification number sewn to it so that I don’t forget which one is which.
A screenshot of the many, many knots I have yet to attend to
Studio shot
The 6 paper knots are those that appear in several of my finished photographs
Studio shot
The Line-up
This illustrates the elements of the Klein bottle. The bottle has one continuous surface only, like the two Moebius strips that join to make it. There is no real inside, you can’t fill it up. Topology doesn’t allow surfaces to intersect so instead of the neck of the bottle poking through the belly, it has to step into 4-space to bypass an approaching intersection. The idea is quite mind-boggling. It’s not the fourth dimension of time and space, which is also mind-boggling,. Topologists tell me 4-space is a dimension at a right-angle to our 3-D world.
Sketchbook braid for knot 10_79
A braid is a diagram indicating the crossings and the order of the crossings of a knot. Each knot has a number which indicates how many times the knot crosses itself and a second underscored to indicate its unique number. So this braid is of a knot with ten crossings and it is the 79th in the ten crossing category. I believe there are 179 knots altogether with ten crossings.
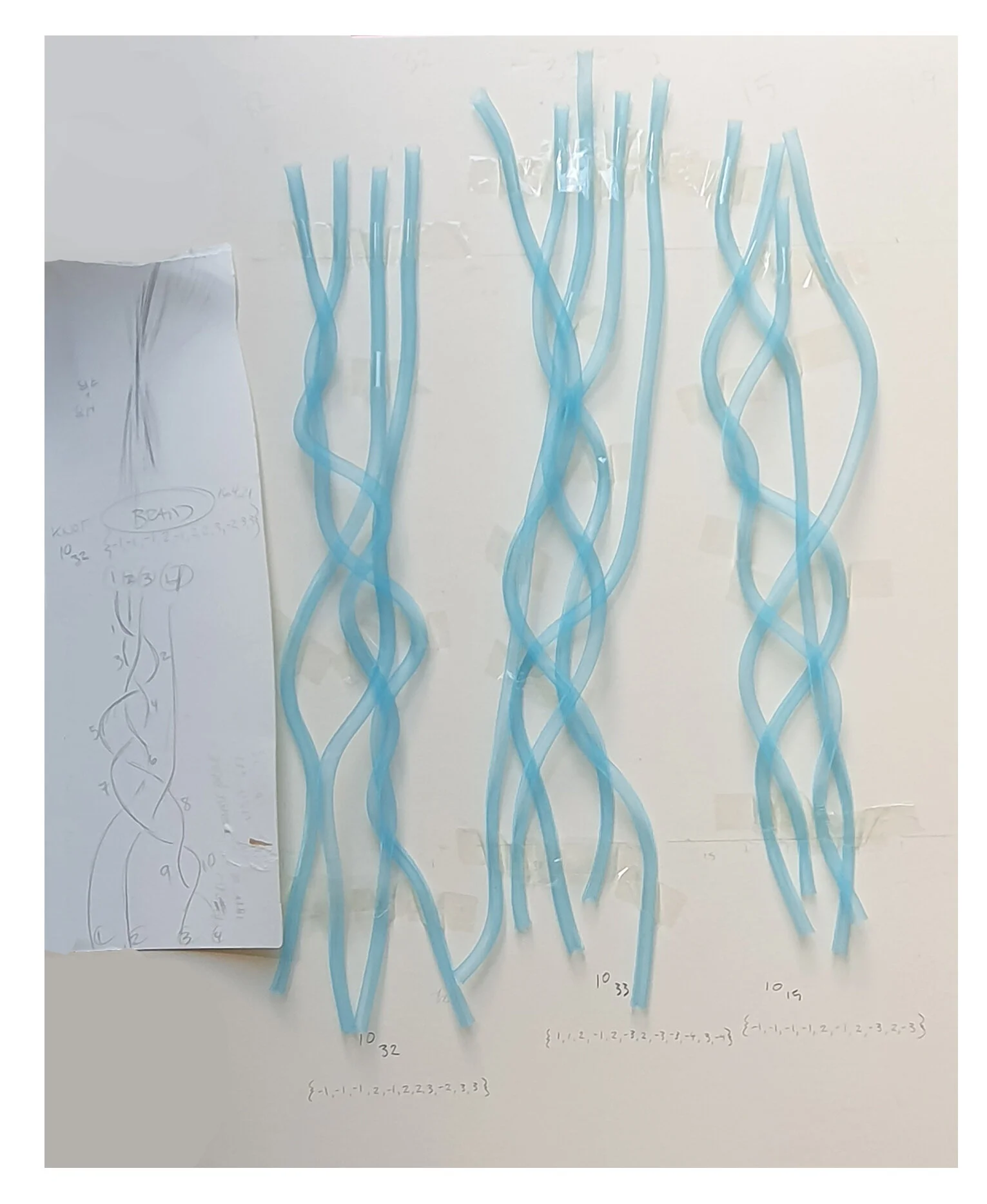
braids for knots 10_15, 10_33 and 10_32
I frequently use the device of planes dividing the image. It locates the subject firmly in the space and give a counterbalancing rigidity to the flowing imagery.
braids for knots 10_32 and 9_7
I am trying to find a way to represent topological braiding that accords with my sense of the rogue tendencies of living things when confined by structure.
Sketchbook
Braids are really a single continuous thread that describes the order of the crossings. each knot has. Topological knots are closed. Unlike a shoelace you can tie a knot in and then undo, these knots have their ends fused together. In a braid you can follow the thread all the way around and end up where you started.
Notebook
My topologist father in the centre of my pin-board